Problem of the Week
Problem C and Solution
Colour by Numbers
Problem
Jessica is going to colour the hexagons in the tiling below. Each hexagon will be coloured with a single colour. If two hexagons share a side, then they will be coloured with a different colour. What is the fewest number of colours that Jessica can use to colour all the hexagons?
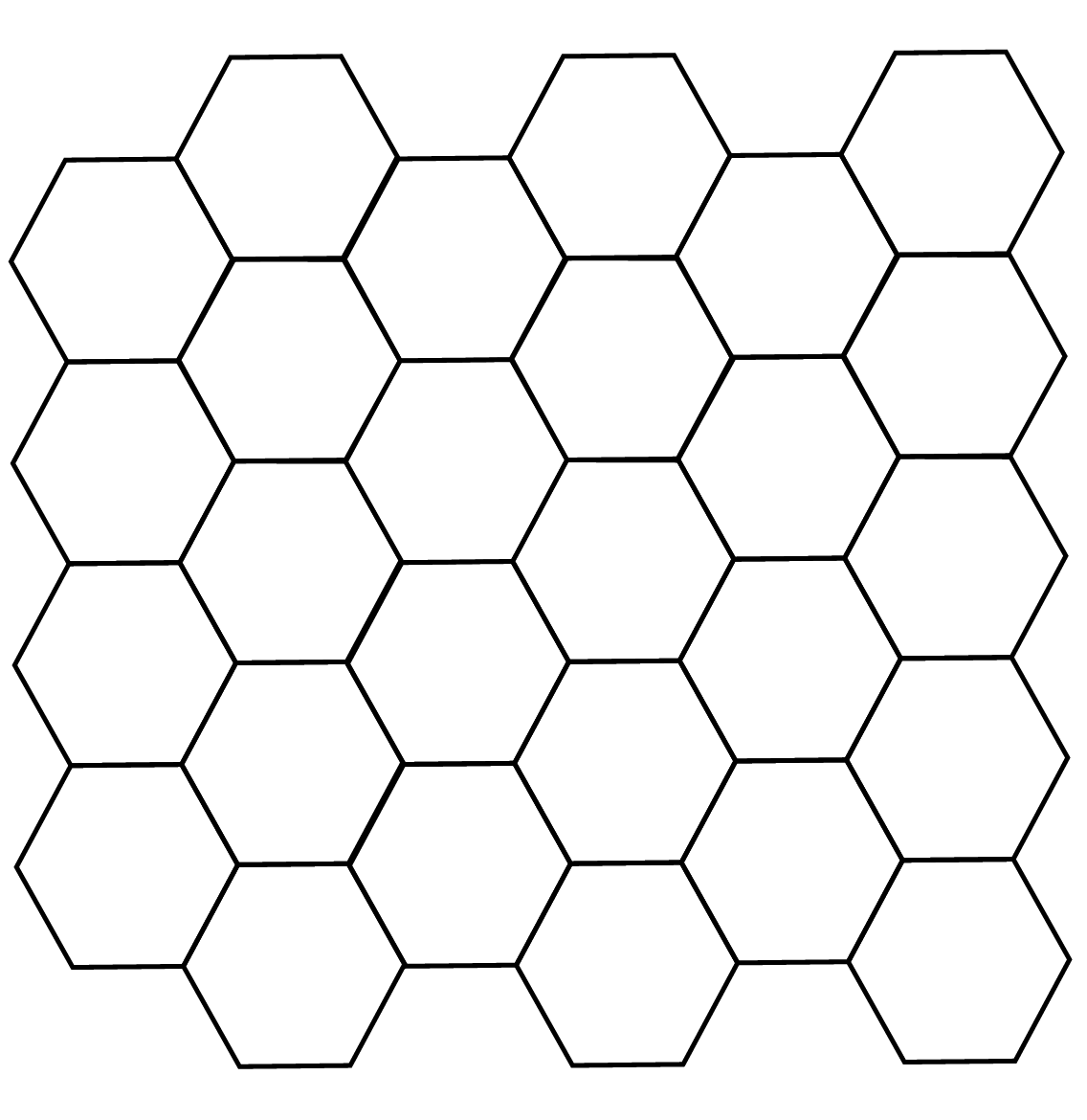
Not printing this page? You can colour the hexagons on our interactive worksheet.
Solution
We first determine if it is possible for her to use only two colours (using just one colour is not possible). We will use the numbers 1, 2, 3 to represent distinct (different) colours. We begin by choosing any group of three hexagons in which each pair of hexagons share a side, as shown. We colour two of the hexagons with colours 1 and 2 (since they share a side). Each of these two coloured hexagons shares a side with the third hexagon, which therefore cannot be coloured 1 or 2.
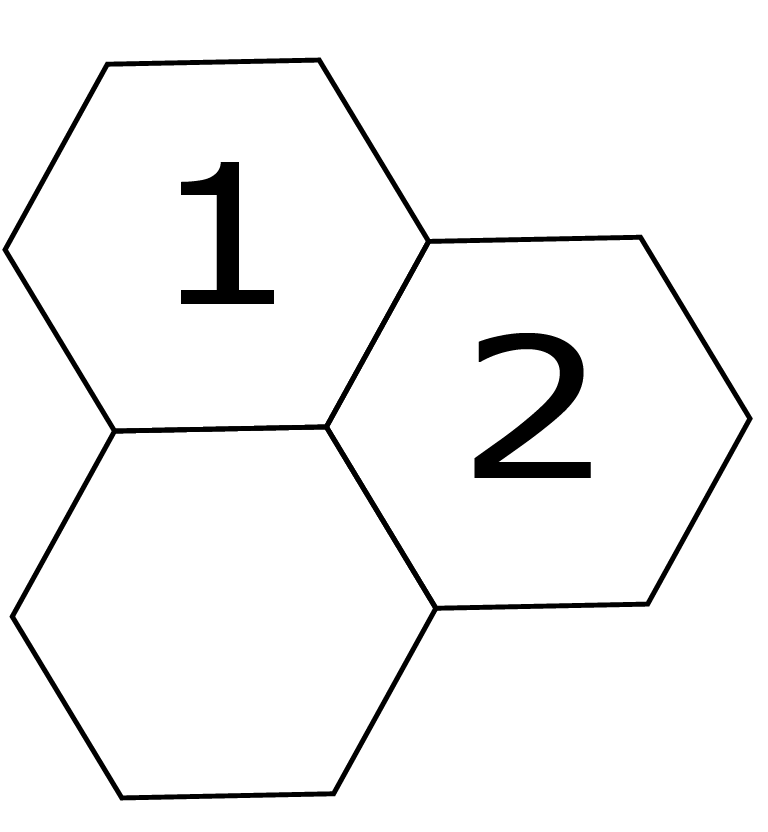
Thus, the minimum number of colours that Jessica can use is at least three.
Next, we determine if the entire tiling can be coloured using only three colours. Here is one possible colouring of the hexagons that uses only three colours.

While other colourings of the hexagons are possible, we can see that it is possible for Jessica to use only three colours and ensure that no two hexagons that share a side are the same colour.
Therefore, the fewest number of colours that Jessica can use to colour all the hexagons is three.
There are many nice patterns of the colours in this tiling. Can you find a different colouring of the tiles that uses only three colours?
