Problem of the Week
Problem D and Solution
The Area Within

Problem

Quadrilateral \(ABCD\) is constructed as follows:

• vertex \(A\) is located at the origin;

• vertex \(C\) is at the intersection point of the lines \(\ell_1:~ 5x+2y=30\) and \(\ell_2:~ x+2y=22\);

• vertex \(B\) is at the intersection point of the \(y\)-axis and \(\ell_2\); and

• vertex \(D\) is at the intersection point of the \(x\)-axis and \(\ell_1\).

Determine the area of \(ABCD\).

Solution

Let the coordinates of \(C\) be \((h,k)\) where \(h\) is the horizontal distance from the \(y\)-axis to \(C\) and \(k\) is the vertical distance from the \(x\)-axis to \(C\).

To find the coordinates of \(D\), let \(y=0\) in \(5x+2y=30\). Therefore, the \(x\)-intercept is 6 and the coordinates of \(D\) are \((6,0)\).

To find the coordinates of \(B\), let \(x=0\) in \(x+2y=22\). Therefore, the \(y\)-intercept is 11 and the coordinates of \(B\) are \((0,11)\).

Two methods are provided to find \(C\), the point of intersection of \(\ell_1\) and \(\ell_2\).

• Solving for \(C\) using the method of substitution:
  Rewrite equation \(\ell_2\) as \(x=22-2y\).

  Substitute for \(x\) in \(\ell_1\) so that \(5(22-2y)+2y=30\). Simplifying, \(110-10y+2y=30\). This further simplifies to \(-8y=-80\) and \(y=10\).

  Substituting \(y=10\) in \(x+2y=22\) gives \(x+20=22\) and \(x=2\).

  The coordinates of \(C\), the point of intersection of \(\ell_1\) and \(\ell_2\), are \((2,10)\). Therefore, \(h=2\) and \(k=10\).

• Solving for \(C\) using the method of elimination:
  \[\begin{aligned} \ell_1: \quad 5x+2y&=30\\ \ell_2: \quad ~x+2y&=22\\ \text{Subtracting, we obtain,} \quad 4x&=8\\ \mbox{Therefore,} \quad x&=2\\\end{aligned}\] Substituting \(x=2\) in \(l_1\), \(10+2y=30\) and \(y=10\). The coordinates of \(C\), the point of intersection of \(\ell_1\) and \(\ell_2\), are \((2,10)\). Therefore, \(h=2\) and \(k=10\).

Quadrilateral \(ABCD\) can be divided into two triangles, \(\triangle ABC\) and \(\triangle ACD\).

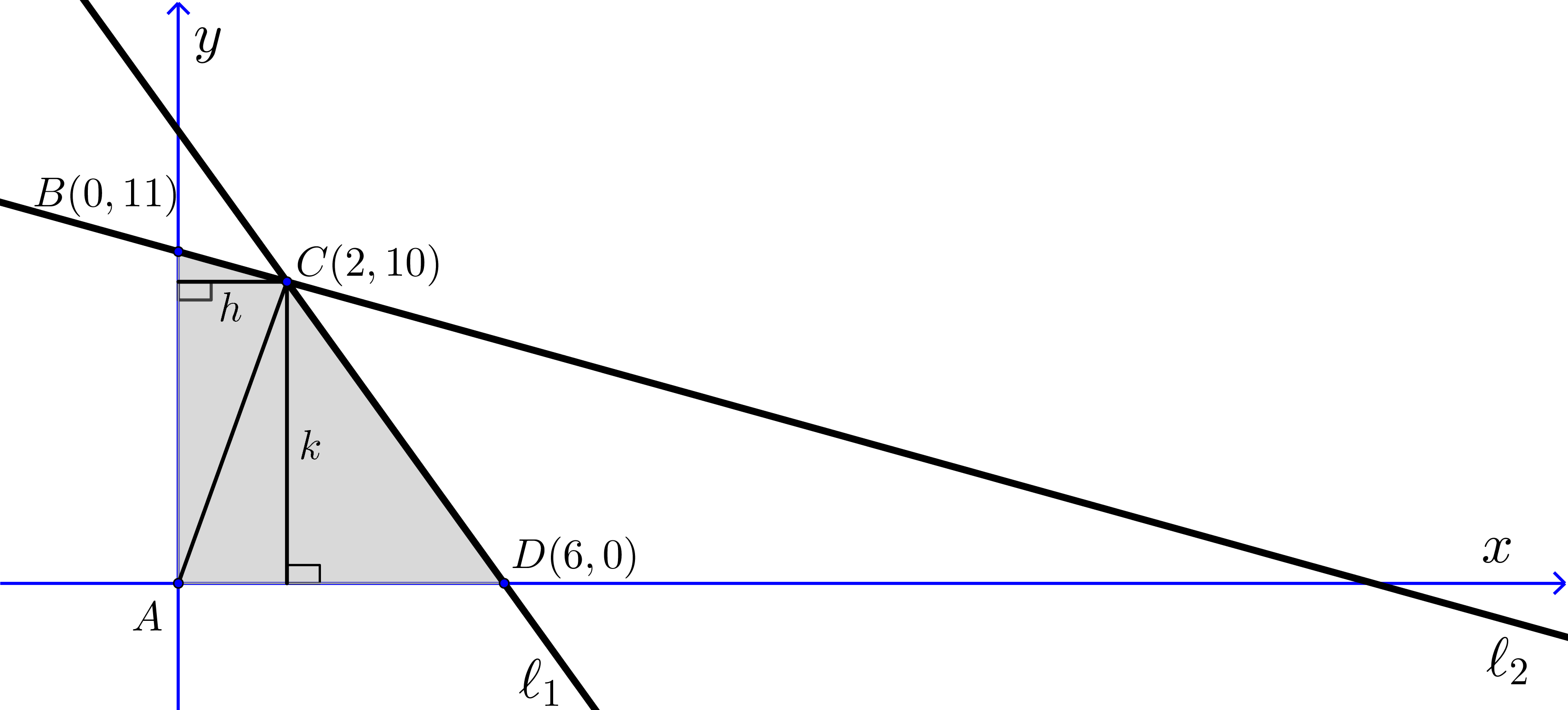
Therefore, \[\begin{aligned} \text{Area } ABCD&=\text{Area }\triangle ABC + \text{Area }\triangle ACD\\ &=\frac{1}{2}(h \times AB) + \frac{1}{2}(k \times AD)\\ &=\frac{1}{2}(2)(11) + \frac{1}{2}(10)(6)\\ &=11+30\\ &=41\end{aligned}\] Therefore, the area of \(ABCD\) is \(41 \text{ units}^2\).