

Problem of the Week
Problem D
Different Lengths
\(\triangle ABC\) is isosceles with \(AB=AC\). All three side lengths of \(\triangle ABC\) and also altitude \(AD\) are positive integers.
If the area of \(\triangle ABC\) is 60 cm\(^2\), determine all possible perimeters of \(\triangle ABC\).
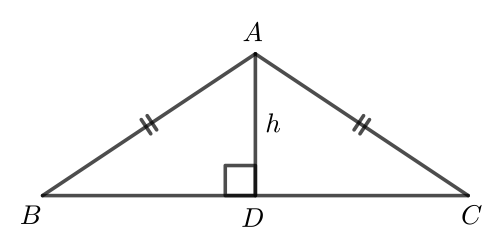
Note: You may use the fact that the altitude of an isosceles triangle drawn to the unequal side bisects the unequal side.