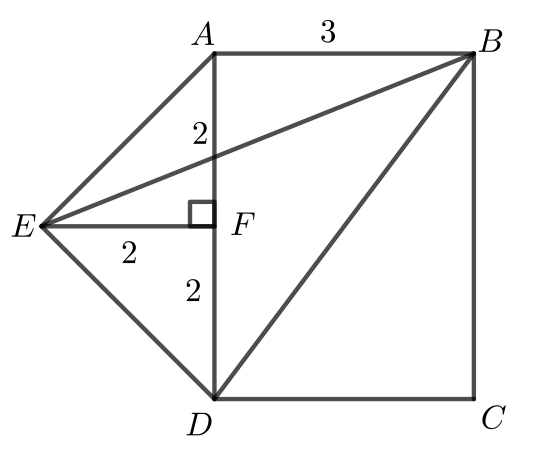
In the diagram, \(ABCD\) is a rectangle. Point \(E\) is outside the rectangle so that \(\triangle AED\) is an isosceles right-angled triangle with hypotenuse \(AD\). Point \(F\) is the midpoint of \(AD\), and \(EF\) is perpendicular to \(AD\).
If \(BC=4\) and \(AB=3\), determine the area of \(\triangle EBD\).

Solution
Since \(ABCD\) is a rectangle then \(AD=BC=4\). Since \(F\) is the midpoint of \(AD\), then \(AF=FD= 2.\)
Since \(\triangle AED\) is an isosceles right-angled triangle, then \(\angle EAD = 45^\circ\).
Now in \(\triangle EAF\), \(\angle EAF = \angle EAD = 45^\circ\) and \(\angle AFE= 90^\circ\).
Since the sum of the angles in a triangle is \(180^\circ\), then \(\angle AEF = 180^\circ - 90^\circ - 45^\circ = 45^\circ\).
Therefore, \(\triangle EAF\) has two equal angles and is therefore an isosceles right-angled triangle.
Therefore, \(EF=AF = 2\).
From this point we are going to look at two different solutions.
Solution 1:
We calculate the area of \(\triangle EBD\) by adding the areas of \(\triangle BAD\) and \(\triangle AED\) and subtracting the area of \(\triangle ABE\).
Since \(AB=3\), \(DA=4\), and \(\angle DAB= 90^\circ\), then the area of \(\triangle BAD\) is \(\frac{1}{2} (3)(4) = 6\).
Since \(AD = 4\), \(EF = 2\), and \(EF\) is perpendicular \(AD\), then the area of \(\triangle AED\) is \(\frac{1}{2} (4)(2) = 4\).
When we look at \(\triangle ABE\) with the base being \(AB\), then its height is the length of \(AF\).
Therefore, the area of \(\triangle ABE\) is \(\frac{1}{2} (3)(2)=3\).
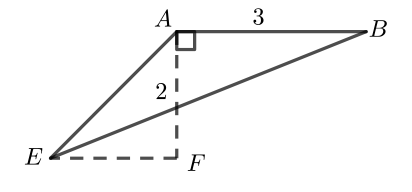
Therefore, the area of \(\triangle EBD\) is \(6 + 4 - 3 = 7\).
Solution 2:
Extend \(BA\) to \(G\) and \(CD\) to \(H\) so that \(GH\) is perpendicular to each \(GB\) and \(HC\) and so that \(GH\) passes through \(E\).
Each of \(GAFE\) and \(EFDH\) has three right angles (at \(G\), \(A\), and \(F\), and \(F\), \(D\), and \(H\), respectively), so each of these is a rectangle.
Since \(AF= EF = FD = 2\), then each of \(GAFE\) and \(EFDH\) is a square with side length 2.
Now \(GBCH\) is a rectangle with \(GB = GA + AB = 2 + 3 = 5\) and \(BC = 4\).
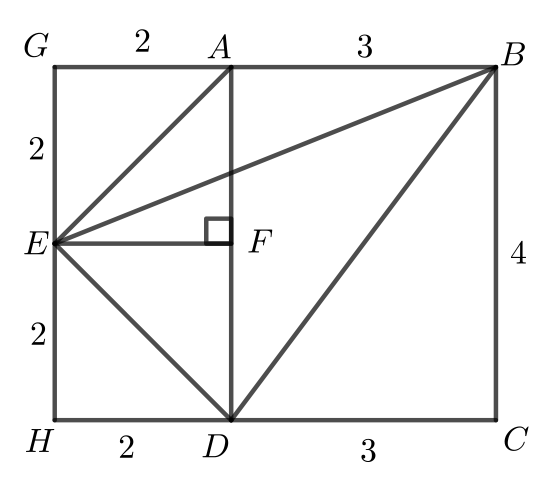
The area of \(\triangle EBD\) is equal to the area of rectangle \(GBCH\) minus the areas of \(\triangle EGB\), \(\triangle BCD\), and \(\triangle DHE\).
Rectangle \(GBCH\) is 5 by 4, and so has area \(5 \times 4 = 20\).
Since \(EG = 2\) and \(GB = 5\) and \(EG\) is perpendicular to \(GB\),
then the area of \(\triangle EGB\) is \(\frac{1}{2} (EG)(GB) = \frac{1}{2}(2)(5)=5\).
Since \(BC = 4\) and \(CD=3\) and \(BC\) perpendicular to \(CD\),
then the area of \(\triangle BCD\) is \(\frac{1}{2} (BC)(CD) = \frac{1}{2}(4)(3)=6\).
Since \(DH = HE = 2\) and \(DH\) is perpendicular to \(EH\),
then the area of \(\triangle DHE\) is \(\frac{1}{2} (DH)(HE)=\frac{1}{2}(2)(2)=2\).
Therefore, the area of \(\triangle EBH\) is \(20 - 5 - 6 - 2 = 7\).
