Melody is building a fence around her beautiful garden. She bought ten wooden posts from Thrifty Buys Used Lumber for the fence. Unfortunately, her bargain posts are all of different lengths.
Using a tape measure, she has found the lengths, in inches, to be: \[57\tfrac{2}{3},~55\tfrac{7}{12},~ 55,~ 56\tfrac{3}{4},~ 57\tfrac{1}{2},~ 55\tfrac{3}{4},~ 56\tfrac{7}{12},~ 57\tfrac{1}{3},~ 56\tfrac{2}{3},~ \text{and}~56\tfrac{11}{12}.\] Now she needs to decide how to build her fence using these posts.
Write the ten lengths of the posts in order from shortest to longest.
Melody decides to adjust the depth of the hole for each post so that all the posts will be the same height above the ground. If she wants all the posts to be \(3\) feet above the ground, what is the deepest hole she will need to dig? It may be helpful to note that \(12\) inches equals one foot.
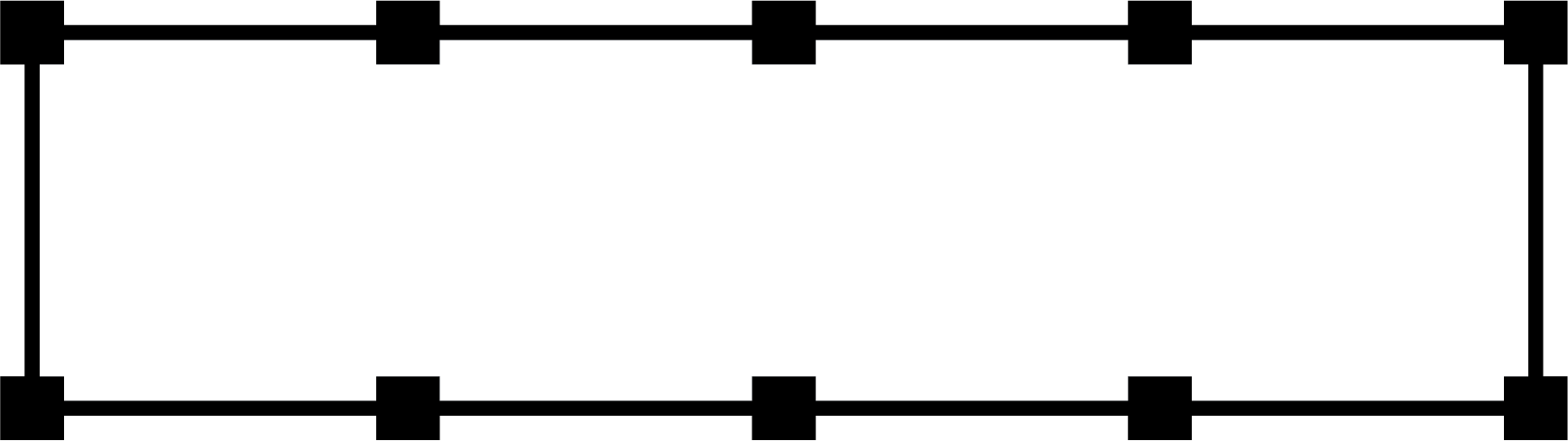
Suppose that Melody’s garden is rectangular. The fence posts are needed in every corner and every \(10\) feet along the fence, as measured from the middle of one fence post to the middle of the next fence post. Draw a diagram for a possible fenced rectangular garden that uses all ten posts. What are the dimensions of your garden, in feet?

The easiest way to compare fractions is to write them with a common denominator. All of the fractions in the post lengths can be converted to an equivalent fraction with a denominator of \(12\) as follows. \[\begin{aligned} &57\tfrac{2}{3}=57\tfrac{8}{12} &56\tfrac{3}{4}=56\tfrac{9}{12} &&57\tfrac{1}{2}=57\tfrac{6}{12}\\ &55\tfrac{3}{4}=55\tfrac{9}{12} &57\tfrac{1}{3}=57\tfrac{4}{12} &&56\tfrac{2}{3}=56\tfrac{8}{12}\end{aligned}\] The lengths of Melody’s posts are then: \[57\tfrac{8}{12},~55\tfrac{7}{12},~ 55,~ 56\tfrac{9}{12},~ 57\tfrac{6}{12},~ 55\tfrac{9}{12},~ 56\tfrac{7}{12},~ 57\tfrac{4}{12},~ 56\tfrac{8}{12},~ 56\tfrac{11}{12}\] Writing these lengths in order from shortest to longest gives: \[55,~ 55\tfrac{7}{12},~ 55\tfrac{9}{12},~ 56\tfrac{7}{12},~ 56\tfrac{8}{12},~ 56\tfrac{9}{12},~ 56\tfrac{11}{12},~ 57\tfrac{4}{12},~ 57\tfrac{6}{12},~ 57\tfrac{8}{12}\] Then we can rewrite the lengths in order from shortest to longest with their original denominators. \[55,~ 55\tfrac{7}{12},~ 55\tfrac{3}{4},~ 56\tfrac{7}{12},~ 56\tfrac{2}{3},~ 56\tfrac{3}{4},~ 56\tfrac{11}{12},~ 57\tfrac{1}{3},~ 57\tfrac{1}{2},~ 57\tfrac{2}{3}\]
The longest post will require the deepest hole. From part (a) we know the length of the longest post is \(57\frac{2}{3}\) inches. Since \(3\) feet equals \(3\times 12=36\) inches, the depth of this hole will be \(57\frac{2}{3}-36=21\frac{2}{3}\) inches.
There are two possibilities for a fenced rectangular garden that uses all ten posts. We need to place one post in each of the four corners of the rectangle, which leaves six posts to place on the sides. The dimensions are calculated by adding up the \(10\) foot spaces between each pair of fence posts.
The first option is a garden with dimensions \(30\) feet by \(20\) feet, as shown.
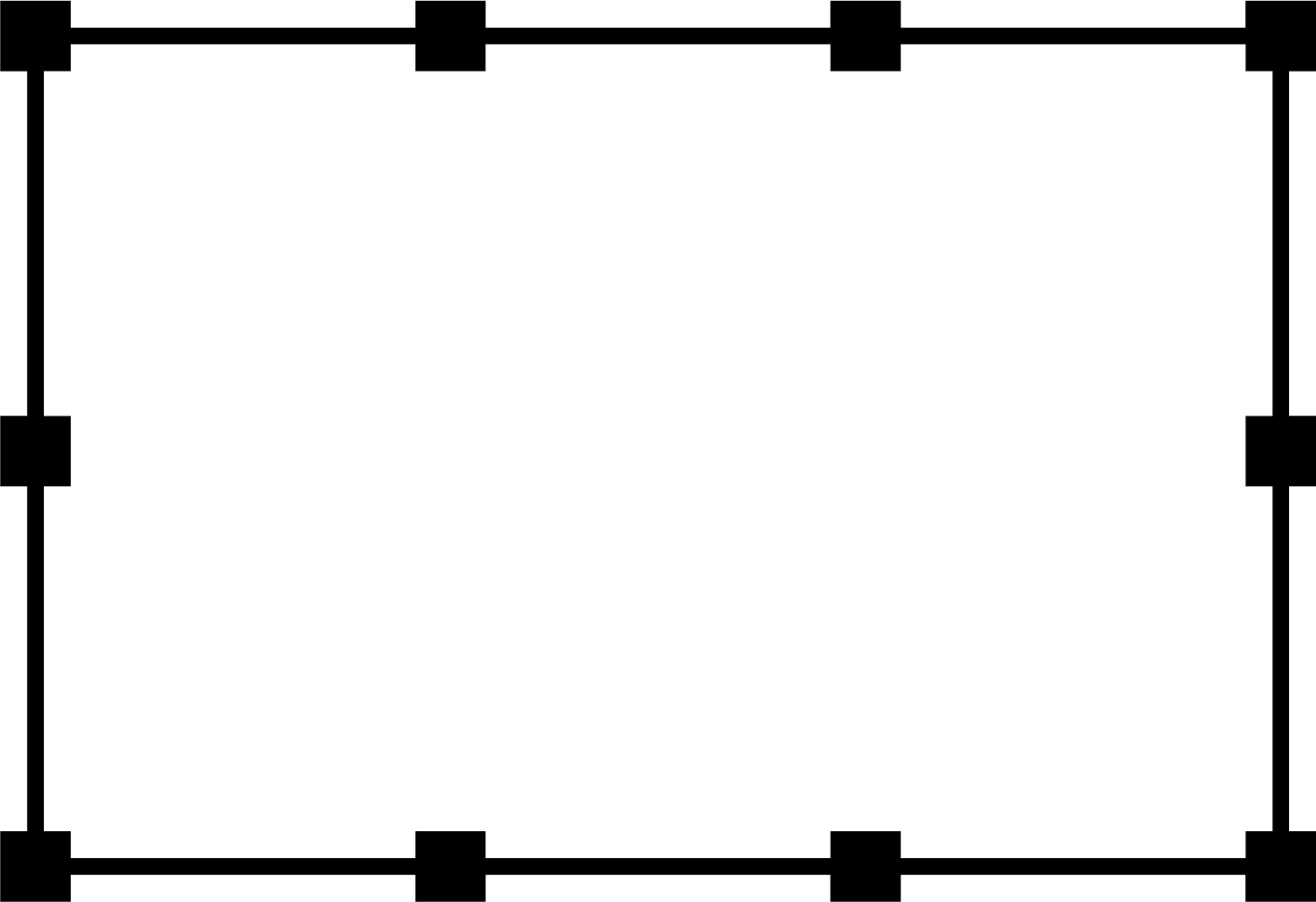
The second option is a garden with dimensions \(40\) feet by \(10\) feet, as shown.