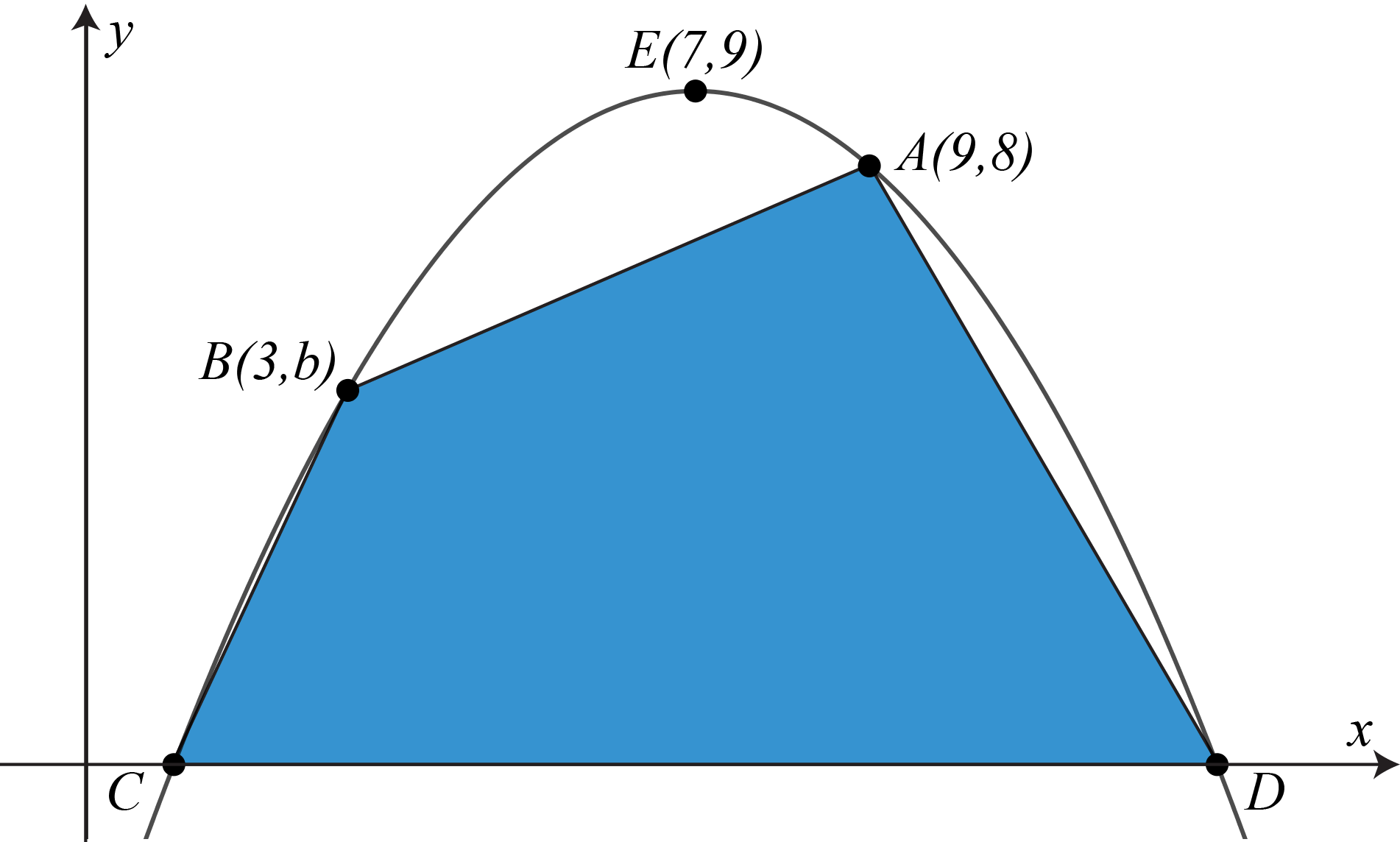

Kenna likes making artistic creations using parabolas, to put on the walls of her math classroom. She drew a parabola with vertex \(E(7,9)\) and plotted points \(A(9,8)\) and \(B(3,b)\) on the parabola as well as points \(C\) and \(D\) where the parabola intersects the \(x\)-axis, with \(C\) to the left of \(D\). Then she connected points \(A\), \(B\), \(C\), and \(D\) to form quadrilateral \(ABCD\), and painted it blue. What is the area of quadrilateral \(ABCD\)?
